Science: Astrophysics:
Problems in
Astrophysics
by Dr.
Kiril P. Panov
Bulgarian
Academy of Sciences
Sofia, Bulgaria
kpanov@astro.bas.bg
In this review, a brief discussion of some astrophysical problems will be given. The review is by no means complete. It points out problems that had significant impact in the recent past and many of them still continue. Let me start with the Sun.
- The Sun: stability of insolation. The solar irradiation on our planet has been very stable at least for the latest one billion years, maybe more. The mere fact that life on Earth has developed and reached such a diversity means that we could make two premises: the orbit of our planet remained stable, and the solar luminosity remained unchanged over hundreds of millions of years. Only a few percent changes in the solar luminosity would have had severe and maybe even fatal consequences on life on Earth. Therefore, looking for a process that could produce the solar energy, one condition it should satisfy is stability over hundreds of millions of years. It is now generally accepted by the astronomical community that hydrogen fusion should be the engine that produces energy in the Sun, as well as in other stars. However, the stability of the hydrogen fusion over long periods of time remains yet to be shown.
- The solar neutrino problem. In the late 1960s, the famous Homestake experiment carried out by R. Davis and J. Bahcall showed deficiency of the detected solar neutrinos. The deficit with respect to the predicted value by the standard theory was later confirmed, the actual value being about one third of the theoretical value. For many years this was a severe problem, putting in doubt the standard solar model. Should the model be changed? May be the fusion reaction temporarily decayed right now? If so, the drop in solar luminosity will be seen after hundreds of years. But then, if this process is unstable, it could have happened in the past, and it did not? Neutrino oscillations were invoked and it seems that this problem is now solved [1-3]. The missing solar electron neutrinos were found to have changed into two other types (muon-type and tau-type) of neutrinos, along the way from the Sun to detectors on Earth. This was experimentally confirmed and it is now generally accepted as a solution of the neutrino problem. Is the problem really closed? Could it be that there would be a different solution leading to the same result?
- The iron rich Sun. Already in the 1970s, O. Manuel, a nuclear chemistry professor at the University of MR pointed out that the Sun has to be iron rich. (www.omatumr.com). He maintains his claim ever since. O. Manuel suggested a scenario where the Sun followed the usual stellar evolution, burned its nuclear fuel and exploded as a supernova (SN). After the SN explosion, the Sun became iron rich star with an iron core. This is the usual scenario by which the standard theory explains the origin of pulsars (neutron stars). Did the Sun really go that far in its evolution? Is it necessary to imply the present evolutionary scenario in order to explain an “iron rich Sun”? I believe, the presence of an iron core in the Sun (if real) could not be expected to be due to evolution according to the standard theory because if the iron core is confirmed, the standard theory would have to be changed. The Sun doesn’t look like a pulsar, indeed, but it could still have an iron core and it is of utmost importance to prove or revoke it. Results from solar seismology provided some information about the interior of the Sun but could this method really penetrate to the solar core? The iron rich Sun, if confirmed, would have an enormous impact on astrophysics, and will probably require changes in the evolutionary models.
- The orbital distances law in planetary systems. The story of the orbital distances law in the Solar system is most remarkable. It started with the Titius – Bode Law (TBL) in the late 18th century, and continued to move the minds for more than two centuries. The TBL predicts the mean distances of the planets from the Sun as:
an = 0.4 + 0.3 . 2n (1)
with “n” being the orbital number. The TBL had initial success in predicting the distances of Uranus and Ceres. Later at about the distance of Ceres a whole belt of asteroids were found. The first failure of the TBL was the discovery of Neptune in 1846 – Neptune was found at much closer distance from the Sun than predicted by the TBL. By the discovery of Pluto in 1930, it has become clear that TBL failed completely. The astronomical community was divided. Many believed (and still do) that the initial success of the TBL was a mere coincidence, a fluke. Others persisted to search for a similar formula that could possibly match better the orbital distances of the planets in the Solar system, but also the exoplanetary systems. There are numerous studies and numerous versions of a TBL – like formula, more or less successful. It seems that the classical TBL predicts well the orbits of the inner planets and works well to the distance of Uranus. It is possible to assume that some kind of orbital distances law really exists, and the TBL was just an approximation to this yet unknown distances law. The same could also be said for all the other suggested formulas up to date – they could be regarded as more or less successful approximations. Recent review could be found in [4-5]. But why is it so important, to look for an orbital distances law for more than two centuries? Because too much is at stake, no doubt. In addition to the possibility of predicting missing planets in studied planetary systems, there are two other important issues, which would follow from an orbital distances formula. The basic assumption of the gravitational collapse that is supposed to have built the planetary systems is that it is a random event in space and time. In the framework of this concept the Solar system should be understood as a series of randomly occurred collapses in the (supposed) primordial cloud of matter. If so, how could it be possible that random events of collapses created regularity in the orbits of planets and satellites – i.e. an orbital distances law? It is an open question. This argument goes with any kind of a distances formula – not just a specific one. The second implication of a distances law concerns the exoplanetary systems. If an orbital distances law exists in the Solar system, it would be natural to expect that a similar law exists also in other exoplanetary systems. Indeed, evidence of a distances law has been found in the systems of 55 Cnc and HD 160691 [5]. But there is another problem in many exolanetary systems, including the two systems mentioned above. Some exoplanets were found so near to the central star that it would be impossible to suppose a gravitational collapse to have occurred there. To deal with this difficulty, it has been suggested that these “close-by” planets originated far away from the respective star but then their orbits have been moved towards the star by friction (or drag) with the environment. This “spiraling down” scenario would be severely put in doubt, if an orbital distances law (whatever the formula may be) exists. Since friction is also a random process, it would be too much of a coincidence to assume that the friction “dragged” the exoplanet and left it at exactly the right place near the star, in order tosatisfy the planetary distances law for this system. In other words, orbital distances law in exoplanetary systems would make the “spiraling down” scenario unbelievable, and the problem with the “close by” planets will remain unsolved. If, on the other hand, these close-by exoplanets did originate in these same places we find them now, we would have to consider a different scenario for the origin of planets.
- The linear density equation for stars and planets. In recent studies [6], it has been shown that a relation exists:
ρ = 3/(8π) . (c2/G) . (1/r2) . (rgr/r) (2)
with ρ, r, rgr being the density, radius, and gravitational radius, and G and c being the gravitational constant and the velocity of light, respectively. This formula was first used for quasars and stars [6], but it seems that it is possible to use it also for planets and for the largest satellites in the Solar system. Eq (2) could be replaced by a linear density equation by introducing the reduced density, which is the density reduced to some radius of choice. If I take r = 8 . 1013 cm, as radius to which all densities are reduced [6], eq (2) turns to:
ρ~ = 0.251549 . rgr/r (3)
with ρ~ being the reduced density. This is a simple linear equation with respect to rgr/r , easy to fit to stars, or planets, or satellites. It turns out that the fit is satisfactory for the stars B0 – M5 , shown in Fig (1). In a similar way one can obtain the fit of eq. (3) for the 9 big planets and for 19 largest satellites of the Solar system. This is a new finding, but it raises new and serious problem. The application of eq (3) for all these structures means that there is a common relation between radius, mass, and reduced density, and which applies for different structures as stars, planets and satellites. On the other hand, the theory of gravitational collapse believed to explain the origin of stars, planets and satellites, is a
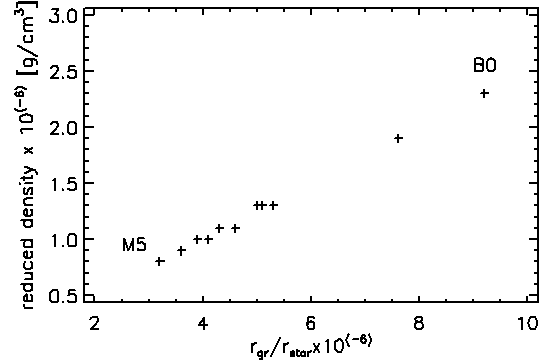
Fig (1). The linear relation of stellar
reduced density (to a radius of 8. 1013 cm)
with the stellar rgr/rstar. The sequence
is: B0, B5, A0, F0, A5, F5, G5, G0, K0, M0, M5. The linear equation is: ρ~ = -2. 10-9 +
0.2505 . rgr/rstar
(courtesy of The Open Astronomy Journal)
random process, as mentioned above. How was it possible to build all these structures in a random process and still have the relation (3) fulfilled? Unless this problem is solved, we would have to re-consider and possibly abandon the theory of gravitational collapse for the origin of stars, planets and satellites. Below we shall see that the same problem concerns also quasars.
- The problem of local quasars. For more that 40 years continues the dispute about the nature of quasars. The dispute is based on the interpretation of their large redshifts, unprecedented in astrophysics. The majority of professional astronomers believes that the large redshifts of quasars are indicators of their cosmological distances in an expanding Universe. Because of their large (cosmological) distances, quasars have to be extremely bright – the brightest objects in the Universe.[7-9] . The only physical process known that could provide the necessary luminosity for quasars, according to this model is the accretion onto a huge black hole. There is also a minority view that maintains that quasars (at least some of them) could be of local origin. Prominent proponents of the local quasar concept are Margaret and Geoffrey Burbidge, Halton Arp etc. Local origin means that quasars are at about the distances to low redshift galaxies, which might have ejected them. It was suggested that local quasars have been ejected by some active galaxies of low redshift [10-15]. If so, the large redshifts (or at least a major part of the redshift) have to have different, non-cosmological origin. Many different causes for non-cosmological redshifts were suggested. In [16-17] it has been considered the gravitational reddening in quasars’ redshifts as a possible origin, which was also corroborated in [6].
There is substantial evidence for the scenario of Arp [18-19], which was developed also in [6], and which assumes that ejected quasars evolve when receding from the parent galaxy and become finally small mass companion galaxies. From this scenario, a number of crucial problems arise and they are listed below:
- Local quasars seem to have been ejected by active nuclei of galaxies. How were they ejected? The known physical processes seem to be insufficient to explain this ejection.
- Local quasars seem to be single bodies of large masses and dimensions, and close to their respective gravitational radius. A theory of such large bodies does not yet exist.
- The physical process releasing enormous energy in local quasars is presently unknown. For local quasars, the accretion model onto a black hole does not work.
- Local quasars seem to evolve with decreasing density, but increasing dimensions and luminosities [6]. They seem to build stellar populations around them at some stage of their evolution that could become low mass companion galaxies. How this happens is a mystery at present.
- In some quasars, high abundances of “metals” were found. This is unexpected and seems very strange in view of the predictions of the standard theory. According to the theory, the only way to produce heavy elements is provided by in late stages of stellar evolution. On the other hand, quasars should be very young objects, if their redshifts are cosmological. This difficulty remains also in the local quasar concept.
- The Karlsson sequence of preferred values for quasars’ redshifts [20-22] has been long standing problem. It now seems that only the gravitational components of quasars’ redshifts follow the Karlson sequence but it is still not yet clear how the Karlsson sequence could be obtained by the evolution of quasars [6].
- Local quasats also seem to obey the linear density relation, eq.(3). Fig (2) shows a sample of 341 local quasars, taken from [6]. This shows a possible link between local quasars, stars and even planets. It may be that the link exists in their origin, but how?
- For local quasars, a relation “density – luminosity” was found [6]. Fig (3) shows this relation. Apparently, increasing density in quasars results in faint luminosity. This might help us to understand another long-standing problem: - the existence of hidden (dark) matter in the Universe. It is presently believed that the dark matter in the Universe is about 23%.
- Local quasars concept has been regarded as a contradiction to the expansion of the Universe, now generally accepted. It was shown [6] that this is not so. In all quasars’ redshifts, there is a cosmological component, due to the expansion of the Universe. Local quasars do not contradict the theory of expanding Universe (the Big Bang theory).
- If this evolutionary scenario for local quasars is confirmed, the end product of evolution of local quasars should be low mass companion galaxies. This seems to lift the difficulties about the gravitational collapse scenario as origin, mentioned above. However, a number of new problems arise, and all these problems may be dealing with one fundamental problem: a disintegration of some primordial dense matter. It is important to note, that about 60 years ago Victor Ambartsumian [23] put forward the idea of disintegration of yet unknown dense matter in stars and in active galaxies. In view of recent developments, the possible implementation of the Ambartsumian’s hypothesis needs to be re-considered seriously.
- If local quasars evolve into companion galaxies, it would be natural to ask a question, how did the large (parent) galaxies originate? The assumption that large (parent) galaxies have been built by a gravitational collapse onto some previously existing kernels seems to stand in conceptual contradiction with the subsequent process of ejecting local quasars, which then may be evolving into companion galaxies.
If we could properly answer all these questions, we would probably have a whole new astrophysics.
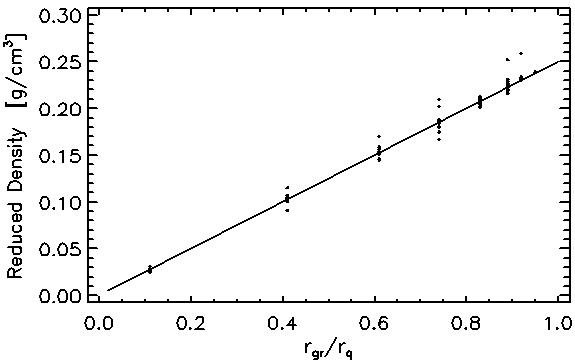
Fig (2). The linear relation of quasar
reduced density with rgr/rq for the sample of 341 QSOs. The mean line equation is: ρ˜ =
0.0002 + 0.251 . rgr/rq
(courtesy of The Open Astronomy Journal).
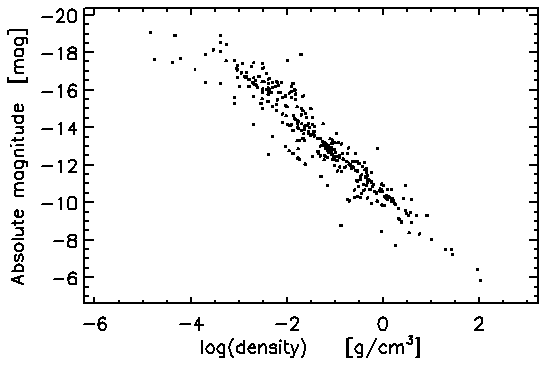
Fig (3).
Relation “absolute magnitude – density” for 341 sample quasars.
(courtesy of The Open Astronomy Journal).
7. The mystery of the “dark matter”.
In 1933, Fritz Zwicky studied the orbital velocities of galaxies in clusters and postulated the “missing mass”. It has turned out that orbital velocities of galaxies are too large for the observed masses of these galaxies and the clusters of galaxies could not be stable, unless there is a “hidden, dark mass” in these galaxies. It is this dark mass that prevents the cluster of galaxies from flying apart. So began the story of the “dark matter”, which is unresolved to this present day. The next chapter of this story refers to studies of the rotational velocities of spiral arms in galaxies. In 1939, Horace Babcock reported results of the rotational curve of the Andromeda galaxy and he found that mass-to-luminosity ratio increases with galactic radius. But the real alarm about the flat rotational curves in galaxies came from the studies of Vera Rubin in the 1970s. She (and collaborators) found [24] that the flat rotational curves are quite common and very different from the expected decrease of rotational velocity with increasing galactic radius. The decreasing orbital velocity of stars at large distances from the galactic center is expected if stars are moving on Keplerian (stable) orbits. Vera Rubin postulated again the need of introduction of some “dark, invisible matter”, and which has to be distributed in the halo of the galaxy. Without such a dark matter, the spirals of galaxies would be unstable, i.e. the stars in these spirals should fly apart. There are alternative suggestions that try to avoid the dark matter introduction. However, these alternatives are even more radical. One such hypothesis is based on possible departure from the Newtonian dynamics (Modified Newtonian Dynamics – MOND). This is a very radical idea, indeed. There may be others, not less exotic hypothesis, which would leave at least the Newtonian dynamics intact? The Vera Rubin – Ford – effect (flat rotational curves) is unquestionable and the flat rotational curves of galaxies are crucial evidence that needs to be explained. But are we sure that spiral arms are really dynamically stable? Maybe they are not. Stability of the galaxy as a whole follows from the gravitational collapse theory for the origin of galaxies. How the spiral arms originated seems to be yet uncertain. If galaxies originate in a different process – not in a gravitational collapse - maybe, spiral arms could not be stable? Could they be the result of activity of the galactic nucleus in the past? The spirals could have been ejected from the galactic nuclei, as suggested by Halton Arp? Then the spiral arms could be dynamically unstable and in due time, spiral arms (i.e. the stars that form it) could fly apart and spirals would be destructed sometime in future. Only these stars in a spiral arm that have the “right” Keplerian velocity for the respective distance from the nucleus would remain in stable orbits. This possibility could not be excluded. However, it raises new problems. The basic one is: how do galaxies originate? What kind of activity of the galactic nucleus is responsible for the building of the spiral arms?
There is now evidence coming from the study of local quasars (see Fig (3)).
It may, or may not have a bearing on the dark matter problem. This diagram shows that quasars with higher density are less luminous. If confirmed, it could be that large quantities of matter in the Universe are not luminous because of high density. This could open a new direction to solve the problem of dark matter. However, if so, we should look for dark matter in the nuclei of galaxies, not in their halos.
8. The “black holes” problem. Black holes are now believed to exist in central
regions (nuclei) of galaxies, may be in all galaxies. A black hole was discovered in our galaxy too, a most exciting discovery indeed. Black holes are believed to “swallow” in-falling matter that could never appear again. The accretion of matter onto black holes releases energy. It is the “engine”, believed to produce the energy in quasars, according to the cosmological model for quasars. Since nothing can escape from a black hole, not even the light, the presence of a black hole can be noticed only by its gravitational field and its influence on moving stars in the surroundings of the black hole. There is a fundamental difficulty about black holes that is usually omitted in discussions. We actually don’t know what a black hole is. The in-falling matter that crosses the border of the gravitational radius could not be stopped and will “fall down” forever. Does this mean, we would be dealing with a body (black hole) with a “zero” radius and a density that reaches infinity? In the framework of the present theory, there is no escape from such a conclusion. On the other hand, my simple physical reasoning revolts against such a conclusion. What if our theory breaks down and would have to be replaced in the cases of black holes, i.e. for bodies with “very high density”? If this is the case, then the in-falling of matter will have to stop at some point in the black hole, when density reached some “very high value”, and due to yet unknown repulsive forces. By all means, a black hole is a very different state of matter and its properties may be revealed by a deeper understanding of the sub-atomic physics of matter.
9. The “white hole” modification of the “black hole” concept.
The possibility of introduction of new physics to study high density structures makes it possible to inverse the concept of black holes. We could also invoke the concept of “white holes”. A white hole should in principal be the same strange high density structure like a black hole. The difference would be that a white hole does not need to accrete matter in order to produce energy. The energy could be produced inside the white hole by yet unknown processes, possibly due to disintegration of the high density matter. We could also speculate that somehow in a white hole the border of the gravitational radius could be crossed from the inside to outside, releasing energy and possibly also matter in the surroundings. This concept may seem radical and incomprehensive but the problems with the black holes are as much incomprehensive and clearly show that we need new concepts. One possible implication of the white holes could be the quasars.
10. The acceleration of the expansion of
the Universe. The “dark energy”.
The first direct evidence for the existence of “dark energy” came from supernovae observations in distant galaxies [25-26]. It has been found that the expansion of the Universe was accelerated during the last 5 billion years. In order to account for the acceleration, the concept of “dark energy” was introduced, which is the most popular at present. Dark energy is a mysterious feature of space and remains a matter of speculations. There is another possible argument for introducing dark energy. Observations show that the Universe is very nearly “flat”, i.e. the average density of the Universe should be about 10-29 g/cm3. The amount of “usual” matter (~4%) and dark matter (~23%) are not enough to attain this critical density. We would need yet another ~73% of mass, and which is introduced as “dark energy”. (The famous equation E = mc2 provides the link between mass and energy). Dark energy should act as a negative (repulsive) pressure, and should not influence the gravitational attraction between masses. No need to be concerned about the fate of the Solar system, neither about the other gravitationally bound structures. It is interesting to note that the first attempt to introduce dark energy was done by A. Einstein. He believed that the Universe has to be static and introduced a “repulsive term” in his equations, in order to balance gravity. This is the famous “cosmological constant” which prevents the Universe from gravitational contraction. Effectively, it was introduction of dark energy. Later on the observations of Edwin Hubble showed that the Universe is expanding. In the following decades, there was no need of the cosmological constant. Was it really a failure, as Einstein admitted? In the 1970s, Alan Guth proposed a negative (repulsive) pressure field, similar to the dark energy, which caused an exponential expansion of the Universe shortly after the Big Bang. In view of the recent discovery of accelerated expansion, the concept of the cosmological constant was revived as one possible cause for the acceleration. There are other interpretations of the dark energy and this matter is far from closed. From the interpretation of the dark energy, the ultimate fate of the Universe could possibly be predicted. Maybe after the expansion the Universe will be contracting again towards a Big Crunch? Or, may be, the Universe will go on expanding forever?
11. Is there an alternative to the gravitational collapse theory?
During the past decades, the origin of stars, planetary systems, and galaxies has been explained by a gravitational collapse of primordial clouds of matter. This theory is widely supported and it can be found in every textbook. It should be noticed, however, that there is no direct evidence for a gravitational collapse in the Universe and all the evidence available is indirect. In such cases, with only circumstantial evidence, one is allowed to admit that also different solution of the problem of origin of structures may be possible, and every single possibility has to be checked out. I mentioned above that observational evidence in quasar studies shows effects of possible disintegration of some dense primordial matter. Problems were pointed out with the Sun, with planetary systems, quasars and galaxies, and all these problems have the same basic root – the assumption of the gravitational collapse as origin of these structures. The alternative could be the hypothesis of Victor Ambartsumian, so far totally neglected in astrophysics. It seems possible to try to resolve the problem of origin of galaxies, stars, and planets using the concept of disintegration. Dealing with such an intricate problem, some speculations seem inevitable.
Let me start with the Big Bang. The whole Universe we know emerged from a “very dense” state of matter. It may have been an act of creation, or it was a repetition of a cycle – a new phase of expansion? It could have been the first stage of the disintegration of the primordial dense matter, and then the remnants of the Big Bang continued to further disintegrate in successive stages (cascades). At some stage, the large galaxies may have been built, than these galaxies ejected quasars, which produced companion galaxies in the next stage of disintegration. Going down the “ladder” of this “cascade”- disintegration”, at some “stair” of this “ladder”, the stars may have been built, than the next two “stairs” down would be the planets and their satellites. The guiding evidence for a “cascade-disintegration” scenario should be the density of structures. Density of structures should be decreasing all the way down the “ladder” of disintegration. Presently, there is not much evidence of this process to reach a compelling conclusion. It may be instructive though to compare the densities of the largest satellites in the Solar system with the densities of the inner planets (densities of the giant planets are very much affected by their mighty atmospheres). For a long time it has been a puzzle, why is the Moon density (~3.3 g/cm3) less than the density of the Earth (~5.5 g/cm3)? This seems to be the general trend for densities of the satellites, which are in the range of ~3.5 g/cm3 (Io) to ~0.98 g/cm3 (Tethys). This is much less than the densities of the inner planets: 5.5 g/cm3 (Earth) to 3.9 g/cm3 (Mars). If the hypothesis of “cascade disintegration” is correct this difference in density would be a natural consequence of going down the “ladder” of disintegration from planets to satellites, i.e. one “stair” below.
Future studies may bring more evidence to this picture. It should be said that there are many signs for important changes coming to astrophysics.
Vera Rubin said once, quote: “in a very real sense, astronomy begins anew”.
Indeed, very actual words.
References
[1] Pontecorvo B. Mesonium and anti-mesonium. Zh Eksp Teor Fiz 1957; 33: 549-551.
[2] Pontecorvo B. Neutrino Experiments and the Problem of Conservation of Leptonic Charge. Zh Eksp Teor Fiz 1967; 53: 1717.
[3] Ahmad QR, et al. Measurement of the rate of interactions produced by 8B solar neutrinos at the Sudbury Neutrino Observatory. Phys Rev Letters 2001; 87: 071301.
[4] Nieto MM. The Titius – Bode Law of Planetary Distances: Its History and Theory, 1972, Oxford, Pergamon Press.
[5] Panov K. The Orbital Distances Law in Planetary Systems. OAJ 2009; 2: 90-94.
[6] Panov K. Study of Possible Local Quasars and Search for a Quasar-Stellar Connection. III. A Sample of 341QSOs. OAJ 2013; 6: 20-47.
[7] Antonucci R. Unified models for active galactic nuclei and quasars. AR Astron Astrophys 1993; 31:473-521.
[8] Djorgowski SG, et al. The origins and the early evolution of quasars and supermassive black holes. Proceed. of XI Marcel Grossmann meeting on General Relativity 2008.
[9] Kembhavi AK, Narlikar JV. Quasars and Active Galactic Nuclei: an introduction. Cambridge University Press, Cambridge 1999.
[10] Burbidge EM, Burbidge GR, Solomon PM, and Strittmatter PA. Apparent associations between bright galaxies and quasi-stellar objects. Astrophys. J 1971; 170: 233-240.
[11] Arp HC. Catalogue of discordant redshift associations. APEIRON 2003, Montreal, Canada.
[12] Arp HC. Quasars, Redshifts, and Controversies. Interstellar Medium 1987,
Berkeley, USA.
[13] Burbidge G. The reality of anomalous redshifts in the spectra of some QSOs
and its implications. Astron Astrophys 1996; 309: 9-22.
[14] Burbidge GR. Noncosmological Redshifts. Publ Astron Soc Pacific 2001; 113: 899-902.
[15] Hoyle F, Burbidge G. Anomalous redshifts in the spectra of extragalactic objects. Astron Astrophys 1996; 309: 335-344.
[16] Hoyle F, Fowler WA. Gravitational Red-shifts in Quasi-Stellar Objects. Nature 1967; 213: 373-374.
[17] Das PK. Physical properties of collapsed objects with large central gravitational redshifts. Mon Not RAS 1976; 177: 391-408.
[18] Arp H. Quasar creation and evolution into galaxies. J Astrophys Astron 1997; 18: 393-406.
[19] Arp H. Evolution of quasars into galaxies and its implications for the birth and evolution of matter. APEIRON 1998; 5: 135-142.
[20] Karlsson KG. Possible discretization of quasar redshifts. Astron Astrophys 1971; 13: 333-335.
[21] Karlsson KG. On the existence of significant peaks in the quasar redshift distribution. Astron Astrophys 1977; 58: 237-240.
[22] Burbidge G, Napier WM. The distribution of redshifts in new samples of Quasi-Stellar objects. Astron J 2001; 121: 21-30.
[23] Ambartsumian VA. La structure et l’evolution de l’univers. 11th Conseil de Physic Solvay, 1958, Bruxelles.
[24] Rubin VC, Ford WKJr. Rotation of the Andromeda Nebula from a Spectroscopic Survey of Emission Regions. Astrophys J 1970; 159: 379-403.
[25] Ries AG, et al. Observational Evidence from Supernovae for an accelerating Universe and a Cosmological constant. Astron J 1998; 116: 1009-1038.
[26] Perlmutter S, et al. Measurements of Omega and Lambda from 42 high-Redshift Supernovae. Astrophys J 1999; 517: 565-586.
[ BWW Society Home Page ]
© 2013 The Bibliotheque: World Wide Society